Answer:
( 9 ,0)
Explanation:
Given :
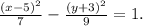 is shifted to the right by 4 units and upward by 3 units
is shifted to the right by 4 units and upward by 3 units
To find : New center of the hyperbola .
Solution : We have given
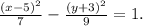
Center of hyperbola is ( 5 , -3)
By the transformation rule f(x) →→ f(x -h) + k it mean f(x) is shifted to right by h unit and k unit up.
Then Center of hyperbola is shifted to the right by 4 units and upward by 3 units.
( 5 , -3) →→ (5 + 4 , -3 + 3
( 5 , -3) →→ ( 9 ,0)
Therefore, new center is ( 9 ,0).