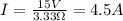1) Total resistance
the total resistance of a circuit with n resistors in parallel is given by
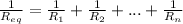
where
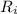
are the single resistances.
In our circuit, we have 3 resistors of
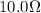
each, so the total resistance of the circuit is given by
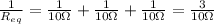
which means a total resistance of

2) Current in the circuit
The current in the circuit can be found by using Ohm's law:

where V is the voltage of the battery and Req is the total resistance we found before. By using V=15 V, we find