Answer:
Worker B needs 9 days to finish the rest of the work
Explanation:
Proportions
Worker A finishes a project on his own in 12 days and worker B does the same in 16 days.
Worker A does 1/12 of the project in one day and worker B does 1/16 of the project in one day. When working together, they do
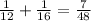
After 3 days they have completed:
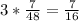
parts of the project, this means it still remains:
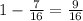
parts of the project and it is done by B alone. Since B does
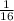 per day, he now takes
per day, he now takes
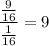
days to complete the project.
Worker B needs 9 days to finish the rest of the work