Question

Find the compound interest on 5000 naira for 2years at 10% per annum​

Answer 1

The compound interest after 2 years of loan is $1,050 .

Explanation:

Given as :

The principal value = p = $5000

The rate of interest = r = 10%

The time period for the loan = t = 2 years

Let the compound interest = C.I

Now, From Compound Interest method

Amount = Principal ×
`(1+(\textrm rate)/(100))^(\textrm time)`

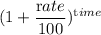
Or, A = p ×
`(1+(\textrm r)/(100))^(\textrm t)`

Or, A = $5000 ×
`(1+(\textrm 10)/(100))^(\textrm 2)`

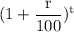
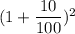
Or, A = $5000 × (1.1)²

Or, A = $5000 × 1.21

Or, A = $6,050

So, The Amount after 2 years = A = $6,050

Now, Again

Compound Interest = Amount - Principal

So, C.I = A - p

Or, C.I = $6,050 - $5000

∴ C.I = $1,050

So, The compound interest = C.I = $1,050

Hence,The compound interest after 2 years of loan is $1,050 . Answer