Question

A baseball player bunts a ball down the first base line. It rolls 32 ft at an angle of 25degrees with the first base path. The​ pitcher's mound is 60.5 ft from home plate. How far must he travel to get to the​ ball

Answer 1

32.3 feet

Explanation:

Let BC=32 feet

AC=60.5 feet

The angle between side BC and AC=45-25=20 degrees

We have to find the distance traveled by player to get to the ball.

Cosine law:
`c^2=a^2+b^2-2ab Cos\theta`

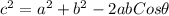
Using Cosine law

`c=\sqrt{(32)^2+(60.5)^2-2(32)(60.5)Cos 20^(\circ)}`

`c=√((32)^2+(60.5)^2-2(32)(60.5)(0.9396))`

`c=32.3 feet`

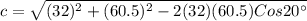
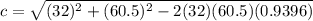
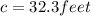
Hence, he travel 32.3 feet to get to the ball.