Answer:
C
Explanation:
Given:
Number of samples, n = 100
let number of sample that favoured candidate A be x = 80
probability, p' = x/n = 80/100 = 0.8
probability, p for significance = 75% = 0.75
Using Z-proportion test statistics, we have
Z =

Substituting the values, we have
Z =
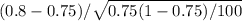
Z = 0.05 / 0.0433 = 1.1547
The p-value is
P(Z>1.1547) = 1 - P(Z≤1.1547)
Checking the Z-score table, P(Z≤1.1547) = 0.8789
Hence, we have 1 - 0.8789
= 0.1241
Hence, the p-value is greater than 0.05, so we cannot say it is very significant.
Hence, the population in favour of candidate A is not significantly greater than 75%