Question

A triangle has side lengths of 7 7 inches, 12 12 inches, and c inches. Enter values to write an inequality that describes the possible values for c , the length of the third side of the triangle

Answer 1

A triangle has side lengths of 7 inches, 12 inches, and c inches. Enter values to write an inequality that describes the possible values for c, the length of the third side of the triangle.

Answer:

The inequality is:
`5 < c < 19`

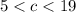
Length of third side "c" can have values greater than 5 but less than 19

Solution:

Given that,

Length of two sides of triangle are 7 inches and 12 inches respectively

Let the length of third side be "c"

The Triangle Inequality Theorem, states that, the sum of the lengths of any two sides of a triangle is greater than the length of the third side

So we get a inequality as:

Case 1:

Sum of length of two sides of triangle > length of third side

`7 + 12 > c`

`19 > c`

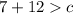
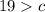
Rewrite,

`c < 19`

Case 2:

Let 12 inches be the length of third side

Sum of sides of length 7 and c > 12

`7 + c > 12\\\\c > 12 - 7\\\\c > 5`

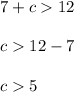
Therefore from case 1 and case 2,

`c > 5 \text{ and } c < 19`

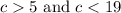
Which can be combined,

`5 < c < 19`

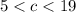
Therefore the possible values of "c" are:

"c" can have values greater than 5 but less than 19