Question

Assume that a​ firm's marginal cost is​ $10 and the elasticity of demand is minus2. We can conclude that the​ firm's profit-maximizing price is approximately

A. ​$20.
B. ​$10.
C. ​$5.
D. The answer cannot be determined without additional information.

Answer 1

Option A. $20

Step-by-step explanation:

Marginal cost be MC, marginal revenue be MR and . We know that

MR = ∆TR ÷ ∆Q

or

MR = (P∆Q+Q∆P) ÷ ∆Q

Here,

P is Profit-maximizing price

or

MR = (P∆Q ÷ ∆Q) + (Q∆P ÷ ∆Q)

or

MR = P + (Q∆P ÷ ∆Q)

we can also write the above equation as

MR =
`P + P((Q)/(P))((\Delta P)/(\Delta Q))`

also,

Price elasticity of demand PED =
`((Q)/(P))((\Delta P)/(\Delta Q))`

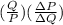
or

MR = P + [ P ÷ (PED) ]

We know MR = MC

Therefore,

MC = P + [ P ÷ (PED) ]

(P − MC) ÷ P = −1 ÷ PED

Substituting the values provided in the question

MC = $10

PED = -2

we get

P = [ PED ÷ (1 + PED)] × MC

P = ( -2 ÷ -1) × 10

or

P =$20

hence,

Option A. $20