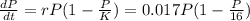Answer:
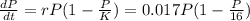
Explanation:
The logistic function of population growth, that is, the solution of the differential equation is as follows:

We use this equation to find the value of r.
In this problem, we have that:
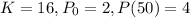
So we find the value of r.

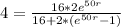
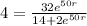
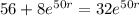


Applying ln to both sides of the equality
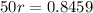
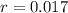
So
The differential equation is