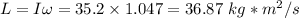Answer:
The angular momentum is
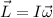
So, we need to find the moment of inertia of the barbell.
The barbell consist of three parts, two masses of 25 kg and a rod of 15 kg.
The moment of inertia of the barbell can be found by summing the moment of inertia of the rod and two masses.
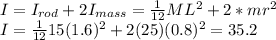
We need to convert 10 rpm to rad/s.
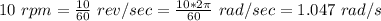
The angular momentum can now be calculated.