Question

If the coefficient of determination is 0.25 and the sum of squares residual is 180, then what is the value of SSY?

A. 60
B. 180
C. 240
D. 800

Answer 1

And then
`SSY=SS_(total)=\sum_(j=1)^n (y_j-\bar y)^2 =240`

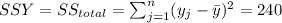
C. 240

Explanation:

Previous concepts

Analysis of variance (ANOVA) "is used to analyze the differences among group means in a sample".

The sum of squares "is the sum of the square of variation, where variation is defined as the spread between each individual value and the grand mean"

When we conduct a multiple regression we want to know about the relationship between several independent or predictor variables and a dependent or criterion variable.

If we assume that we have
`k` independent variables and we have
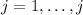`j=1,\dots,j` individuals, we can define the following formulas of variation:

`SS_(total)=\sum_(j=1)^n (y_j-\bar y)^2`

`SS_(regression)=SS_(model)=\sum_(j=1)^n (\hat y_(j)-\bar y)^2`

`SS_(error)=\sum_(j=1)^n (y_(j)-\hat y_j)^2 =180`

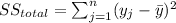
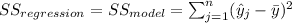
And we have this property

`SST==SSY=SS_(regression)+SS_(error)=SSR+180`

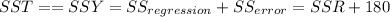
If we solve for SSR we got:

`SSR= SSY-180` (1)

And we know that the determination coefficient is given by:

`R^2 = (SSR)/(SSY)`

We know the value os
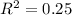`R^2= 0.25` and we can replace SSR in terms of SSY with the equation (1)

`R^2 =0.25= (SSY-180)/(SSY)= 1-(180)/(SSY)`

And solving SSY we got:

`(180)/(SSY)=1-0.25=0.75`

`SSY= (180)/(0.75)=240`

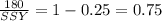
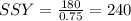
And then
`SSY=SS_(total)=\sum_(j=1)^n (y_j-\bar y)^2 =240`

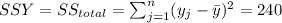
C. 240