Question

A high-jump athlete leaves the ground, lifting her center of mass 1.8 m and crossing the bar with a horizontal velocity of 1.4 m/s. At what minimum speed must she leave the ground to accomplish this?

Answer 1

The minimum speed when she leave the ground is 6.10 m/s.

Step-by-step explanation:

Given that,

Horizontal velocity = 1.4 m/s

Height = 1.8 m

We need to calculate the minimum speed must she leave the ground

Using conservation of energy

`K.E+P.E=P.E+K.E`

`(1)/(2)mv_(1)^2+0=mgh+(1)/(2)mv_(2)^2`

`(v_(1)^2)/(2)=gh+(v_(2)^2)/(2)`

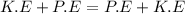
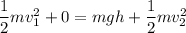
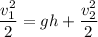
Put the value into the formula

`(v_(1)^2)/(2)=9.8*1.8+((1.4)^2)/(2)`

`(v_(1)^2)/(2)=18.62`

`v_(1)=√(2*18.62)`

`v_(1)=6.10\ m/s`

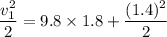
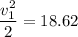
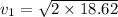
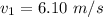
Hence, The minimum speed when she leave the ground is 6.10 m/s.