Answer:
The ball lands in about 3.29 seconds.
The given equation is:
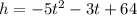 .
.
Explanation:
I do believe the equation is meant to be:
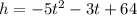 .
.
We want to find
 such that
such that
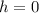 since we want to find the time when the ball has hit the ground.
since we want to find the time when the ball has hit the ground.
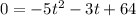
Our objective here is to solve this equation using the quadratic formula,
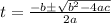 .
.
First we need to compare the equation we have to
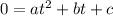 .
.
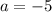
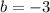


Simplify the denominator first:
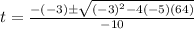
Simplify the inside of the square root:
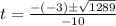
Simplify the -(-3):

So we have either
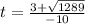 or
or
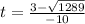 .
.
Let's both of those into our calculator.
 or
or
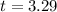
So the ball lands in about 3.29 seconds.