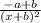Answer:
Yes, you are right.
See explanation.
Explanation:
The definition of derivative is:
 .
.
We are given
 .
.
Assume
 are constants.
are constants.
If
 then
then
 .
.
Let's plug them into our definition above:

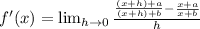
I'm going to find a common denominator for the main fraction's numerator.
That is, I'm going to multiply first fraction by
 and
and
I'm going to multiply second fraction by
 .
.
This gives me:
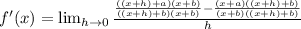
Now we can combine the fractions in the numerator:
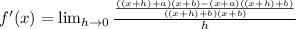
I'm going to multiply a bit on top and see if there is anything than can be canceled:

Note: I do see that
 .
.
I also see
 .
.
I will also distributive in other places in the mini-fraction's numerator.
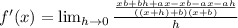
Note: I see
 .
.
I also see
 .
.
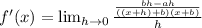
In the numerator of the mini-fraction on top the two terms contain a factor of
 so I can factor that out.
so I can factor that out.
This will give me something to cancel out across the main fraction since
 .
.


So we now have gotten rid of what would make this over 0 if we had replace
 with 0.
with 0.
So now to evaluate the limit, that is also we have to do now.
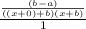
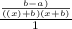
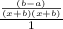
I'm going to go ahead and rewrite this so that isn't over 1 anymore because we don't need the division over 1.

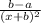
or what you wrote: