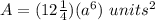Answer:
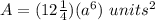
Explanation:
we know that
The area of a square is equal to
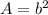
where
b is the length side of the square
In this problem we have
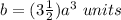
Convert mixed number to an improper fraction

substitute
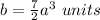
substitute in the formula of area
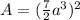
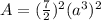
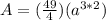
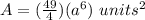
Convert 49/4 to mixed number
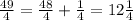
substitute