Answer:
c. 4.1 s
Explanation:
We have been given that a car drives horizontally off a 83-m-high cliff at a speed of 25 m/s . Ignore air resistance.
To solve our given problem, we will use free fall formula. The free fall formula states that the distance the object falls, or height, h, is 1/2 gravity times the square of the time falling.
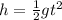
Solve for t:
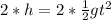
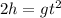
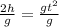
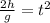
Switch sides:
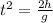
Take positive square root:
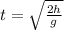
In our given situation
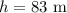 . We know
. We know
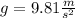 .
.
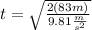
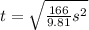

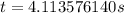
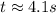
Therefore, it will take 4.1 seconds the car to hit the ground and option 'c' is the correct choice.