Answer:
A) $ 456.85
B) 0.25% per month
C) $ 429.812
Step-by-step explanation:
We solve for the quota of an annuity of 10,000 dollar over two year with a discount rate of 6% compounded monthly
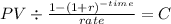
PV 10,000
time 24
rate 0.0075
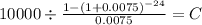
C $ 456.847
b) we need to remove the inflation premium to the 9% compounded monthly
0.09/12 - 0.005 = 0.0075 - 0.005 = 0.0025
c) we should discount this at the real rate
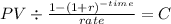
PV 10,000
time 24
rate 0.0025
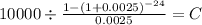
C $ 429.812