Question

A square steel bar has a length of 7.4 ftft and a 2.2 inin by 2.2 inin cross section and is subjected to axial tension. The final length is 7.40339 ftft . The final side length is 2.19966 inin . What is Poisson's ratio for the material?

Answer 1

To proceed to solve this problem we will use the relationships given for longitudinal and lateral strain. This relationship is given by the change in the initial and final length for each of the directions, so the longitundinal strain would be

`\epsilon_x = (l_f-l_i)/(l_i)`

`\epsilon_x = (7.40339-7.4)/(7.4)`

`\epsilon_x = 0.0004581`

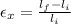
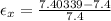
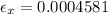
The lateral strain would be

`\epsilon_y = (a_f-a_i)/(a_i)`

`\epsilon_y = (2.19966-2.2)/(2.2)`

`\epsilon_y = -0.000154`

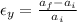
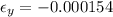
Calculating the Poisson's ratio

`\upsilon = -(\epsilon_y)/(\epsilon_x)`

`\upsilon = -(-0.000154)/(0.0004581)`

`\upsilon = 0.336171`

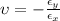
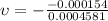
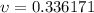
Therefore the Poisson's ratio for the material is 0.336171