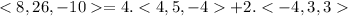Answer:
Explanation:
Linear Combination Of Vectors
One vector
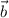 is a linear combination of
is a linear combination of
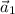 and
and
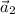 if there are two scalars
if there are two scalars
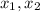 such as
such as
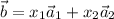
In our case, all the vectors are given in
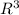 but there are only two possible components for the linear combination. This indicates that only two conditions can be used to determine both scalars, and the other condition must be satisfied once the scalars are found.
but there are only two possible components for the linear combination. This indicates that only two conditions can be used to determine both scalars, and the other condition must be satisfied once the scalars are found.
We have
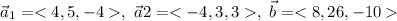
We set the equation
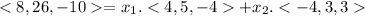
Multiplying both scalars by the vectors
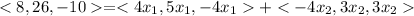
Equating each coordinate, we get
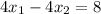
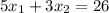

Adding the first and the third equations:
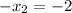
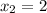
Replacing in the first equation


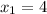
We must test if those values make the second equation become an identity

The second equation complies with the values of
 and
and
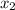 , so the solution is
, so the solution is