Question

Find the minimum value of C=2x+y subject to the following constraints:
Please help me!!

Answer 1

The minimum value of C is 20

Explanation:

we have

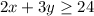`2x+3y\geq 24` ----> constraint A

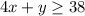`4x+y\geq 38` ----> constraint B

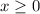`x\geq 0` ----> constraint C

`y\geq 0` ----> constraint D

Using a graphing tool

The solution set of the constraints is the shaded area

see the attached figure

The vertices of the shaded area are (0,38),(9,2) and (12,0)

To determine the minimum value of C substitute the values of x and y of each vertex in the objective function and then compare the results

`C=2x+y`

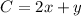
For (0,38) --->
`C=2(0)+38=38`

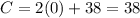
For (9,2) --->
`C=2(9)+2=20`

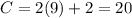
For (12,0) --->
`C=2(12)+0=24`

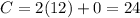
therefore

The minimum value of C is 20