Question

For which value of k are the points (0 -5) (6 k) and (-4 -13) collinear?

Answer 1

Explanation:

Use the 2 points for which you have both an x and y coordinate to write the equation of the line containing those 2 points. When you get the equation, sub in the x value of 6 and solve for y.

Begin by finding the slope of the line:

`m=(-13-(-5))/(-4-0)=(-13+5)/(-4)=(-8)/(-4)=2`

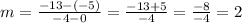
Then use one of the points in point slope form to write the equation in slope-intercept form. I used (0, -5). But either point you choose will give you the exact same line...promise.

y - (-5) = 2(x - 0) and

y + 5 = 2x and

y = 2x - 5

Now sub in the x value of 6 for x and solve for y:

y = 2(6) - 5 and

y = 12 - 5 so

y = 7