Answer:
b. S = 405, D = 0
Explanation:
We have been given that profit for a particular product is calculated using the linear equation:
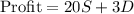 . We are asked to choose the combinations of S and D that would yield a maximum profit.
. We are asked to choose the combinations of S and D that would yield a maximum profit.
To solve our given problem, we will substitute given values of S and D in the profit function one by one.
a. S = 0, D = 0
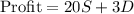
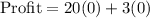
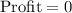
b. S = 405, D = 0
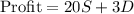
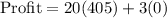

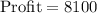
c. S = 0, D = 299
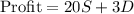
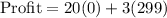
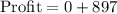

d. S = 182, D = 145
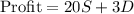
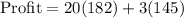
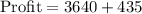
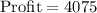
Since the combination S = 405, D = 0 gives the maximum profit ($8100), therefore, option 'b' is the correct choice.