Answer:
$2,385,086
Step-by-step explanation:
To answer this question, we need to use the present value of an ordinary annuity formula:
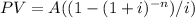
Where:
- A = Value of the annuity
- i = interest rate
- n = number of compounding periods
Because the interest rate is annual, it is convenient to convert it to a monthly rate.
4.5% annual rate = 0.37% monthly rate.
The number of compounding periods will be = 12 months x 30 years
= 360 months
Now, we simply plug the amounts into the formula:
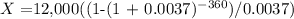
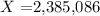
You will need to have saved $2,385,086 if you plan to retire under the aforementioned circumstances.