Question

A linear regression slope coefficient of -2.3 indicates ...

Select one:

a. Researchers would expect a 1 unit decrease in the explanatory variable to be associated with a 2.3 unit increase in the response variable

b. The mean response is 2.3.

c. Researchers would expect a 2.3 unit decrease in the explanatory variable to be associated with a 1 unit increase in the response variable

d. Researchers would expect an average response of 2.3 units when the explanatory variable is 0.

Answer 1

a. Researchers would expect a 1 unit decrease in the explanatory variable to be associated with a 2.3 unit increase in the response variable

True, that's the correct option since for each unit that the explanatory variable increase we have a decrease of 2.3 units for the response variable. And we can interpret it as 1 unit decrease in the explanatory variable we have a 2.3 unit increase in the response variabl.

b. The mean response is 2.3.

False, the slope is not asociated with the mean response.

c. Researchers would expect a 2.3 unit decrease in the explanatory variable to be associated with a 1 unit increase in the response variable

False, The slope is defined as the rate of change of the response variable related to the rate of change for the explanatory variable. The 2.3 (slope) is associated with the response variable and never with the explanatory variable.

d. Researchers would expect an average response of 2.3 units when the explanatory variable is 0.

That's FALSE, when the explanatory variable is 0 we have that
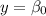`y= \beta_0` and we can associate this value to the slope since is not the correct interpretation.

Explanation:

Let's suppose that we have the following linear model:

`y= \beta_o +\beta_1 X`

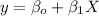
Where Y is the dependent variable and X the independent variable.
`\beta_0` represent the intercept and
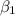`\beta_1` the slope.

In order to estimate the coefficients
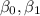`\beta_0 ,\beta_1` we can use least squares estimation.

And for our case
`\hat \beta_1 = -2.3`

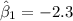
Let's analyze the possible options to select the correct one:

a. Researchers would expect a 1 unit decrease in the explanatory variable to be associated with a 2.3 unit increase in the response variable

True, that's the correct option since for each unit that the explanatory variable increase we have a decrease of 2.3 units for the response variable. And we can interpret it as 1 unit decrease in the explanatory variable we have a 2.3 unit increase in the response variabl.

b. The mean response is 2.3.

False, the slope is not asociated with the mean response.

c. Researchers would expect a 2.3 unit decrease in the explanatory variable to be associated with a 1 unit increase in the response variable

False, The slope is defined as the rate of change of the response variable related to the rate of change for the explanatory variable. The 2.3 (slope) is associated with the response variable and never with the explanatory variable.

d. Researchers would expect an average response of 2.3 units when the explanatory variable is 0.

That's FALSE, when the explanatory variable is 0 we have that
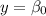`y= \beta_0` and we can associate this value to the slope since is not the correct interpretation.