Question

You have been abducted by aliens and find yourself on a strange planet. Fortunately, you have a meter stick with you. You observe that, if the stick oscillates around one end as a physical pendulum, it has a period of 0.85 s. What is the acceleration of gravity on this planet?

Answer 1

54.6 m/s^2

Step-by-step explanation:

length of the pendulum, l = 1 m

time period of the pendulum, T = 0.85 s

According to the formula of time period of pendulum

`T = 2\pi \sqrt{(l)/(g)}`

`g = (4\pi ^(2)l)/(T^(2))`

`g = (4* 3.14* 3.14* 1)/(0.85* 0.85)`

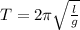
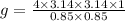
g = 54.6 m/s^2

Thus, the acceleration due to gravity at this planet is 54.6 m/s^2.

Answer 2

`36.4276\ m/s^2`

Step-by-step explanation:

m = Mass of stick

L = Length of stick = 1 m

h = Center of mass of stick =
`(1)/(2)=0.5\ m`

g = Acceleration due to gravity

T = Time period = 0.85 s

Time period is given by

`T=2\pi\sqrt{(I)/(mgh)}`

Moment of inertia is given by

`I=m(L^2)/(3)`

`T=2\pi\sqrt{(m(L^2)/(3))/(mgh)}\\\Rightarrow T=2\pi\sqrt{(L^2)/(3gh)}\\\Rightarrow g=(4\pi^2L^2)/(3hT^2)\\\Rightarrow g=(4\pi^2* 1^2)/(3* 0.5* 0.85^2)\\\Rightarrow g=36.4276\ m/s^2`

The acceleration of gravity on this planet is
`36.4276\ m/s^2`