Question

If an atom of mass 1.5 x 10-25 kg at rest spontaneously emits a photon of wavelength 780 nm, what is the atom's final speed?

Answer 1

To solve this problem it is necessary to apply the concepts related to the conservation of the moment.

The initial moment must be equal to the final moment so that there is balance, that is to say

`P_i = P_f`

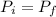
Since there is no velocity at the beginning, the initial moment will be 0, while the moment in the photon must be diminished by the one generated through the wavelength, that is to say

0 = mv - \frac{h}{\lambda}

Recall that h is Planck's constant and λ wavelength, while m is mass and v is velocity, like this:

`0 = 1.5*10^(-25)v-(6.626*10^(-34))/(780*10^(-9))`

`v = (6.626*10^(-34))/(780*1.5*10^(-34))`

`v = 5.6*10^(-3)m/s`

`v = 5.6mm/s`

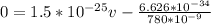
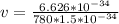
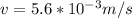
Therefore the atom's final speed is 5.6mm/s