Question

Unpolarized light of intensity Io passes through six successive Polaroid sheets each of whose axis makes a 60 ∘ angle with the previous one. What is the intensity of the transmitted beam?

Answer 1

0.0004883 Io

Step-by-step explanation:

Let Io be the intensity of unpolarised light.

Intensity of light coming out from first polaroid.

I1 = Io / 2

According to law of Malus, the intensity of light coming from the n th polaroid is given by

`I_(n)= I_(1)\left (Cos^(2)\theta  \right )^(n-1)`

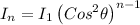
where, n be the number of polaroid and θ be the angle between two polaroid.

`I_(6)= (I_(0))/(2)\left (Cos^(2)60 \right )^(6-1)`

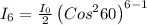
So, I6 = 0.0004883 Io

Thus, the intensity of light coming out from the sixth polaroid is 0.0004883 Io.

Answer 2

`0.00048828125I_0`

Step-by-step explanation:

`I_0` = Unpolarized light intensity

`\theta` = Angle = 60°

Intensity of light through first polarizer

`I_1=(I_0)/(2)`

Intensity of light through nth polarizer

`I=I_1(cos^2\theta)^(n-1)`

when

n = 6

`I_6=(I_0)/(2)(cos^2(60))^(6-1)\\\Rightarrow I_6=0.00048828125I_0`

The intensity of light after passing through the sixth polarizer is
`0.00048828125I_0`