Question

At a time t = 3.10 s , a point on the rim of a wheel with a radius of 0.200 m has a tangential speed of 50.0 m/s as the wheel slows down with a tangential acceleration of constant magnitude 10.3 m/s2 .

Answer 1

51.5 rad/s^2

Step-by-step explanation:

radius, r = 0.2 m

tangential speed, v = 50 m/s

tangential acceleration, a = 10.3 m/s^2

time, t = 3.10 s

The relation between the radial acceeration and the tangential acceleration is given by

a = r x α

where, α is the radial acceleration and r be the radius of the circular path

10.3 = 0.2 x α

α = 51.5 rad/s^2

Thus, the radial acceleration is 51.5 rad/s^2.

Answer 2

Angular acceleration,
`\alpha =51.5\ rad/s^2`

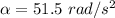
Step-by-step explanation:

It is given that,

Radius of the wheel, r = 0.2 m

Tangential speed of the wheel,
`v=50\ m/s`

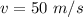
Tangential acceleration of the wheel,
`a_t=10.3\ m/s^2\\`

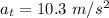
It is assumed to find the angular acceleration of the wheel. It is given by :

`a_t=\alpha * r`

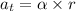
Where

`\alpha` is the angular acceleration of the wheel

So,

`\alpha =(a_t)/(r)`

`\alpha =(10.3\ m/s^2)/(0.2\ m)`

`\alpha =51.5\ rad/s^2`

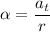
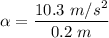
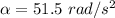
So, the angular acceleration of the wheel is
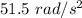`51.5\ rad/s^2`. Hence, this is the required solution.