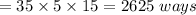Question

For this problem, assume 7 males audition, one of them being Robert, 5 females audition, one of them being Jessica, and 6 children audition. The casting director has 3 male roles available, 1 female role available, and 2 child roles available.

How many different ways can these roles be filled from these auditioners?

Answer 1

Answer:2625 ways

Explanation:

Given

There are 7 males , 5 Females and 6 children

There are 3 males Roles, 1 Female and 2 child roles available

No of ways in which 3 males can be chosen
`=^(7)C_3=35\ ways`

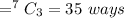
No of ways in which 1 Female can be chosen
`=^(5)C_1=5\ ways`

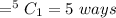
No of ways in which 2 children can be chosen
`=^(6)C_2=15\ ways`

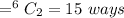
Total no of ways
`=35* 5* 15=2625\ ways`