Question

Which term of the sequence 2,4,6,......512 is 256?​

Answer 1

If this sequence is part of an arithmetic sequence, then its 128-th term would be 256.

Explanation:

The two neighboring terms differ by a constant, 2. As a result, this sequence is likely an arithmetic sequence.

• The first term
  `a_1` is equal to 2.
• The common difference
  `d` (second term - first term) is equal to 2.

The formula for the general
`n`-th term of an arithmetic sequence with first term
`a_1` and common difference
`d` is:

`a_1 + (n - 1) \, d`.

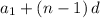In this case, that's equal to

`2 + (n - 1) * 2 = 2 + 2 * (n - 1) = 2\, n`.

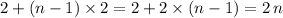Let that expression be equal to
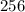`256`. Solve for
`n`:

`2\, n = 256`

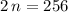
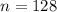`n = 128` (after dividing both sides by
`2`.)

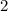Hence, if this sequence is part of an arithmetic sequence, then the 128-th term would be 256.

Answer 2

128

Explanation:

Givens

a = 2

L = 256

d = 2

n = ?

Formula

L = a + (n - 1)*d

Solution

256 = 2 + (n - 1)*2 Subtract 2 from both sides

254 = (n - 1)*2 Divide by 2

254/2 = n-1)*2/2 Do the division

127 = n - 1 Add 1 to both sides..

128 = n