Question

A truck is moving at 25.0 m/s and sees a barrier in the road 100 m ahead. The truck can decelerate at 4 m/s2. Will the truck hit the barrier? If so, when? If not, how close does the truck get to the barrier?

Answer 1

No.

x= 21.875 m .

Step-by-step explanation:

Given that

Speed of the truck ,u = 25 m/s

Deceleration ,a = - 4 m/s²

The distance of barrier from truck ,d= 100 m

The distance travel by truck when driver apply the brake = s m

The final speed of the truck will be zero.

v= 0 m/s

We know that

v²= u² + 2 a s

0²= 25² - 2 x 4 x s

625 = 8 s

s= 78.125 m

The distance s is less than the distance d,Therefore the truck will not hit the barrier.

The distance from the barrier where the truck will be stop ,x= 100 - 78.125 m

x= 100 - 78.125 m

x= 21.875 m

Answer 2

No, the truck will not cross the barrier.

The closeness of the truck to the barrier is of 21.875 m

Solution:

As per the question:

Velocity of the truck, v = 25.0 m/s

Acceleration of the truck, a = - 4
`m/s^(2)`

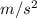
Now,

Since, the barrier at a distance of 100 m. Thus in order to check whether the truck hit the barrier or not, we will see the distance, d it covers by using the kinematic eqn:

`v'^(2) = v^(2) + 2ad`

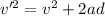
Final velocity, v' = 0 m/s

Initial velocity = v

Now,

`0^(2) = 25^(2) + 2* -4d`

`- 8d = - 625`

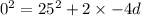
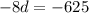
d = 78.125 m

Thus the truck will not cross the barrier.

Distance between the barrier and the truck:

100 - 78.125 = 21.875 m