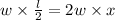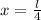Answer:
C. 1/4 the length of the plank from the left end
Step-by-step explanation:
- We consider the beam to be mass-less and having a uniformly distributed load of
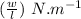
For a beam in equilibrium we have all the forces and moments on the beam in balanced condition.
Mathematically:
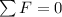
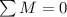
For the forces to be balanced:
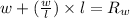
 ..........................(1)
..........................(1)
Taking moment about the left end in the balanced condition:
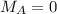
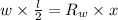
put the value of
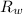 from eq.(1)
from eq.(1)