Question

Water flows in a canal of trapezoidal cross section with a depth of 5 feet and side slopes are at 40 degrees and a bottom width of 12 feet. The bottom drops 1.4 ft per 1000 ft of length. The canal is lined with smooth concrete with a friction coefficient of 0.012. Determine the Froude Number of this channel.

Answer 1

Freoude's number is directly related to the forces of inertia and the Force of Gravity.

To find it we must find the distance x (see in the graph)

then proceed to find how wide is the top that would allow us to find the total area of the object.

From the same geometric figure we will proceed to find the length of the slope and the diameter of the wet.

With the geometric values found it will be possible to find the hydraulic radius the hydraulic depth and the Freude Number.

Let's start:

`tan40 = (x)/(5)`

`x = 4.195ft`

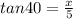
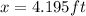
Now the top Width

`width = 2(4.195)+12 = 20.39'`

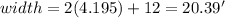
We can find the Area:

`A= (1)/(2) 5 (12+20.39) = 80.97ft^2`

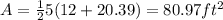
And the Slope Length

`S_l = √(4.195^2+5^2)`

`S_l = 6.52ft`

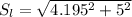
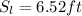
The welted perimeter is twice the slope length plus 12:

`\phi = (2*6.52)+12`

`\phi = 25.05'`

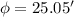
Now the hydraulic radius is given depending on the Area and the perimeter wetted therefore:

`R = (A)/(\phi)`

`R= (80.97)/(25.05)`

`R= 3.23'`

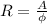
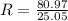
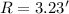
The friction coefficient (n) was given as 0.012 and the bed slope is equal to the proportion between the bottom drops and its length:

`S_o = (1.4)/(1000)`

With this values we can calculate the velocity which is given as,

`V = (1)/(n) R^(2/3)So^(1/2) \Rightarrow (1)/(0.012)*3.23^(2/3)((1.4)/(1000))^(1/2)`

`V= 6.815ft/s`

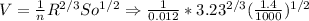
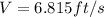
The value of the Hydraulic Depth (D) is equal to the area per Width, then

`D = (A)/(Width) = (80.97)/(20.39)`

`D = 3.97'`

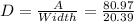
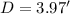
Finally the Froud's number is given in function of the velocity, the gravity and the hydraulic Depth:

`Fr = (V)/(gD^(1/2))`

`Fr = (6.815)/((32.17*3.97)^(1/2))`

`Fr = 0.602`

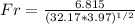
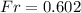
Therefore the Froude Number of this channel is 0.602.