Answer:
1) E = 2.25 i^+ 0.809j^) 10⁹ N / C , 2) E = 2.39 10⁹ N / C , 3) θ = 19.8º , 4) F = 19.12 10⁹ N , 5) E = (1.32 i^+ 3.56 j^) 109 N/C
Step-by-step explanation:
1) The equation for the electric field is
E = k q / r²
Where K is the Coulomb constant that is worth 8.99 10⁹ N m² /C², q is the load and r is the distance of the load to the test point
Since the electric field is a vector magnitude, we can find its component
X axis
Ex = k q / x²
where the distance on the axis is
x = √ (X₂-x₁)²
x = √ (-15 + 9)² = 6 m
Eₓ = 8.99 10⁹ 9/6²
Eₓ = 2.25 10⁹ N /C
Y Axis
y = √ (y₂-y₁)² = √ (15-5)² = 10 m
 = 8.99 10⁹ 9/10²
= 8.99 10⁹ 9/10²
 = 0.809 10⁹ N / C
= 0.809 10⁹ N / C
E = Eₓ i^+
 j^
j^
E = 2.25 i^+ 0.809j^) 10⁹ N / C
2) the magnitude can be found using the Pythagorean triangle
E = √ (Eₓ² +
 ²)
²)
E = √ (2.25² + 0.809²) 10⁹
E = 2.39 10⁹ N / C
3) to find the angle let's use trigonometry
tan θ =
 / Eₓ
/ Eₓ
θ = tan⁻¹
 / Eₓ
/ Eₓ
θ = tan⁻¹ (0.809 / 2.25)
θ = 19.8º
Regarding the positive side of the x axis
4) a charge q2 = 8C is placed, let's calculate the force
F = q E
F = 8 2.39 10⁹
F = 19.12 10⁹ N
5) The total electric field at the origin, let's look for its components
q₁ = 9C
r₁ = -9 i ^ + 5 j ^
q₂ = 8 C
r₂ = -15 i ^ + 15 j ^
X axis
Eₓ = E₁ₓ + E₂ₓ
Eₓ = k q₁ / Dx₁² + k q₂ / Dx₂²
Eₓ = 8.99 10⁹ (9 / (9-0)² + 8 / (15-0)²)
Eₓ = 1.32 109 N / A
Y Axis
 =
=
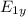 +
+
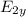
 = k q₁ / Δy₁² + k q₂ / Δy₂²
= k q₁ / Δy₁² + k q₂ / Δy₂²
 = 8.99 109 (9/5² + 8/15²)
= 8.99 109 (9/5² + 8/15²)
 = 3.56 109 N / A
= 3.56 109 N / A
E = (1.32 i^+ 3.56 j^) 109 N/C