Question

Future scientists: Education professionals refer to science, technology, engineering, and mathematics as the STEM disciplines. A research group reported that 28% of freshmen entering college in a recent year planned to major in a STEM discipline. A random sample of 95 freshmen is selected. Round the answer to at least four decimal places. Part 4 of6 Find the probability that the proportion of freshmen in the sample of 150 who plan to major in a STEM discipline is between 0.29 and 0.37 The probability that the proportion of individuals in the sample of 150 who plan to major in a STEM discipline is between 0.29 and 0.37 is

Answer 1

The probability that the proportion of freshmen in the sample of 150 who plan to major in a STEM discipline is between 0.29 and 0.37 is 0.3855

Explanation:

The probability that the proportion of freshmen in the sample of 150 who plan to major in a STEM discipline is between 0.29 and 0.37 can be calculated by finding z-scores and subtracting P(z<z(0.29)) from P(z<z(0.37))

z-score in the binomial distribution of 28% of freshmen entering college in a recent year planned to major in a STEM discipline can be calculated using the equation:

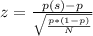`z=\frac{p(s)-p}{\sqrt{(p*(1-p))/(N) } }` where

• p(s) is proportion of freshmen we are interested (0.37, 0.29)

• p is the proportion found in recent year found by research group (28% or 0.28)

• N is the sample size (150)

Then z(0.37)=
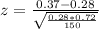`z=\frac{0.37-0.28}{\sqrt{(0.28*0.72)/(150) } }` ≈ 2.4550 and P(z<2.4550)=0.993

z(0.29)=
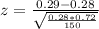`z=\frac{0.29-0.28}{\sqrt{(0.28*0.72)/(150) } }` ≈ 0.2728 and P(z<0.2728)=0.6075

Then P(z(0.29)<z<z(0.37))=0.993-0.6075=0.3855