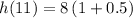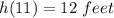Answer:
(a)
![\displaystyle h(t)=y(t)=8\left [ 1+sin\left ( (\pi)/(6)t+(\pi)/(3) \right ) \right ]](https://img.qammunity.org/2020/formulas/physics/college/tpxzm6ksse34ky37upecybvjb48443l3bg.png)
(b) Height at 11:00 a.m. = 12 feet
Step-by-step explanation:
Sinusoidal Function
It refers to a mathematical curve with a smooth and periodic oscillation. Its name comes from the sine function but it can be a cosine function too. They only differ by the phase angle of 90 degrees or
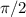 radians.
radians.
The sine function is characterized by
The minimum value is -1 when the argument is
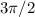 radians or 270 degrees
radians or 270 degrees
The maximum value is 1 when the argument is
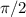 or 90 degrees
or 90 degrees
It completes a full cycle in
 radians (or 360 degrees)
radians (or 360 degrees)
It's zero at 0 and

It repeats itself along infinite cycles with the same characteristics
We need to model the height of the tide above low tide at time t, t expressed in hours from midnight
We know the following data
At 1:00 and 13:00, the tide is high at 16 feet above the low tide, assumed to be 0 m
At 7:00 and 19:00, the tide is low at 0 m.
(a) The general sine function is expressed as
![y(t)=Asin(wt+\phi)+B\ \ .........[1]](https://img.qammunity.org/2020/formulas/physics/college/hjkj8qwlbynmq4yx4g7mcokxsgcedsvait.png)
Where A is the amplitude, w is the angular frequency,
 is the phase, B is the vertical shift
is the phase, B is the vertical shift
We know that at t=1, the sine must be at max (value =1) and at t=7 it must be at min (value=-1). Replacing in [1]
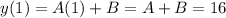
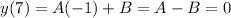
We get these equations
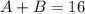
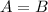
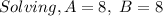
Using the same data as before, when t=1 the argument of the sine must be
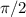 , so it reaches it max, and when t=7, the argument must be
, so it reaches it max, and when t=7, the argument must be
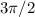 . Then:
. Then:
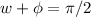
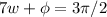
Solving, we get


The function is complete now
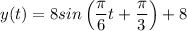
Factoring
![\displaystyle h(t)=y(t)=8\left [ 1+sin\left ( (\pi)/(6)t+(\pi)/(3) \right ) \right ]](https://img.qammunity.org/2020/formulas/physics/college/tpxzm6ksse34ky37upecybvjb48443l3bg.png)
(b) We need to find h when t=11
![\displaystyle h(11)=8\left [ 1+sin\left ( (\pi)/(6)11+(\pi)/(3) \right ) \right ]](https://img.qammunity.org/2020/formulas/physics/college/s3mi2bhugrbxusr01mjf6rw3wa9qdeyapr.png)
![\displaystyle h(11)=8\left [ 1+sin\left ( (13\pi)/(6) \right ) \right ]](https://img.qammunity.org/2020/formulas/physics/college/yupv225lmd0cdr1n3rnz14vdpuw2maono1.png)