Answer:
x =-2t , y = -t, z= t
Explanation:
x+2y+z+3w=0
x-y+w=0
y-z+2w=0
The augmented system would be given by:
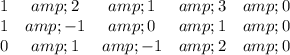
Now we can do operations in order to reduce it to the row echelon form
1) R1 *(-1) + R2
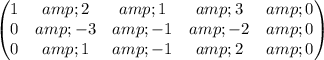
2) R2 *(-1/3)

3) R2*(-1)+R3
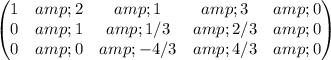
4) R3*(-3/4)
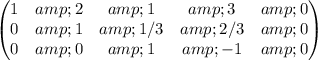
5) R3(-1/3) + R2; R3(-1) +R1
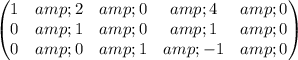
6) R2(-2) +R1
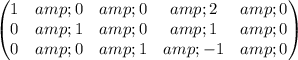
Let w=t a free variable then the solution is given by:
x =-2t , y = -t, z= t