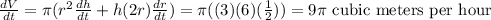Answer:
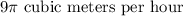
Explanation:
Since, the surface area of a cylinder,
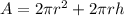 ................(1)
................(1)
Where,
r = radius,
h = height,
If
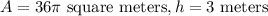
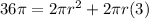

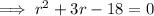
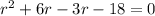 ( by middle term splitting )
( by middle term splitting )
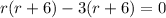
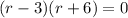
By zero product property,
r = 3 or r = - 6 ( not possible )
Thus, radius, r = 3 meters,
Now, differentiating equation (1) with respect to t ( time ),
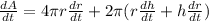
∵ h = constant, ⇒ dh/dt = 0,
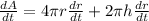
We have,
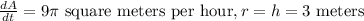
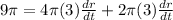
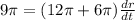

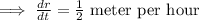
Now,
Volume of a cylinder,
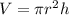
Differentiating w. r. t. t,