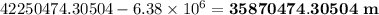Question

A satellite that goes around the earth once every 24 hours iscalled a geosynchronous satellite. If a geosynchronoussatellite is in an equatorial orbit, its position appearsstationary with respect to a ground station, and it is known as ageostationary satellite

Find the radius Rof the orbit of a geosynchronous satellite that circles the earth.(Note that Ris measured from the center of the earth, not the surface.) You mayuse the following constants:
The universal gravitational constant G is 6.67 \times 10^{-11}\;{\rm N \; m^2 / kg^2}.
The mass of the earth is 5.98 \times 10^{24}\;{\rm kg}.
The radius of the earth is 6.38 \times 10^{6}\;{\rm m}.

Answer 1

35870474.30504 m

Step-by-step explanation:

r = Distance from the surface

T = Time period = 24 h

G = Gravitational constant = 6.67 × 10⁻¹¹ m³/kgs²

m = Mass of the Earth = 5.98 × 10²⁴ kg

Radius of Earth =
`6.38* 10^6\ m`

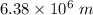
The gravitational force will balance the centripetal force

`(GMm)/(R^2)=m(v^2)/(R)\\\Rightarrow v=\sqrt{(GM)/(R)}`

`T=(2\pi r)/(v)\\\Rightarrow T=\frac{2\pi r}{\sqrt{(GM)/(r)}}`

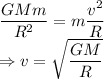
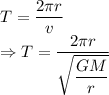
From Kepler's law we have relation

`T^2=(4\pi^2r^3)/(GM)\\\Rightarrow r^3=(T^2GM)/(4\pi^2)\\\Rightarrow r=\left(((24* 3600)^2* 6.67* 10^(-11)* 5.98* 10^(24))/(4\pi^2)\right)^{(1)/(3)}\\\Rightarrow r=42250474.30504\ m`

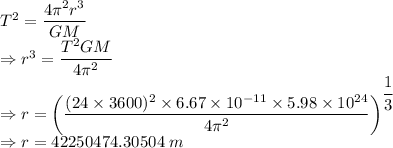
Distance from the center of the Earth would be

`42250474.30504-6.38* 10^6=\mathbf{35870474.30504\ m}`