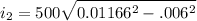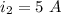Answer:
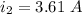
Step-by-step explanation:
LC Circuit
It's a special circuit made of three basic elements: The AC source, a capacitor, and an inductor. The charge, current, and voltage are oscillating when there is an interaction between the electric and magnetic fields of the elements. The following variables will be used for the formulas:
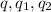 = charge of the capacitor in any time
= charge of the capacitor in any time
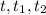
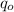 = initial charge of the capacitor
= initial charge of the capacitor
 =angular frequency of the circuit
=angular frequency of the circuit
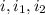 = current through the circuit in any time
= current through the circuit in any time
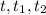
The charge in an LC circuit is given by
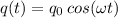
The current is the derivative of the charge

We are given
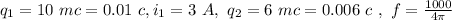
It means that
![q(t_1) = q_0 \, cos (\omega t_1 )=q_1\ .......[eq 1]](https://img.qammunity.org/2020/formulas/physics/college/x3xa2kp9azx9lxbx3jlhvn9mykt9sxcogc.png)
![i(t_1) = - \omega q_0 \, sin(\omega t_1)=i_1.........[eq 2]](https://img.qammunity.org/2020/formulas/physics/college/jj6k48bk0p1wj75u5relc2o0dbmdzasnvr.png)
From eq 1:
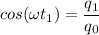
From eq 2:
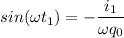
Squaring and adding the last two equations, and knowing that

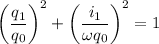
Operating

Solving for
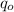

Now we know the value of
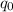 , we repeat the procedure of eq 1 and eq 2, but now at the second time
, we repeat the procedure of eq 1 and eq 2, but now at the second time
 , and solve for
, and solve for
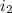
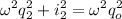
Solving for
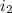

Now we replace the given values. We'll assume that the placeholder is a pi for the frequency, i.e.
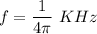
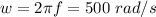
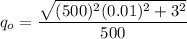
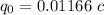
Finally