Question

A function f is​ _____ on an open interval I​ if, for any choice of x1 and x2 in​ I, with 1 less than

Answer 1

If
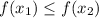`f(x_1)\leq f(x_2)` whenever
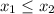`x_1\leq x_2` f is increasing on I.

If
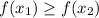`f(x_1)\geq f(x_2)` whenever
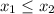`x_1\leq x_2` f is decreasing on I.

Explanation:

These are definitions for real-valued functions f:I→R. To help you remember the definitions, you can interpret them in the following way:

When you choose any two numbers
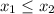`x_1\leq x_2` on I and compare their image under f, the following can happen.

• 
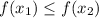  `f(x_1)\leq f(x_2)`. Because x2 is bigger than x1, you can think of f also becoming bigger, that is, f is increasing. The bigger the number x2, the bigger f becomes.

• 
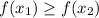  `f(x_1)\geq f(x_2)`. The bigger the number x2, the smaller f becomes so f is "going down", that is, f is decreasing.

Note that this must hold for ALL choices of x1, x2. There exist many functions that are neither increasing nor decreasing, but usually some definition applies for continuous functions on a small enough interval I.