Answer:
Step-by-step explanation:
The amplitude of the oscillation under SHM will be .5 m and the equation of
SHM can be written as follows
x = .5 sin(ωt + π/2) , here the initial phase is π/2 because when t = 0 , x = A ( amplitude) , ω is angular frequency.
x = .5 cosωt
given , when t = .2 s , x = .35 m
.35 = .5 cos ωt
ωt = .79
ω = .79 / .20
= 3.95 rad /s
period of oscillation
T = 2π / ω
= 2 x 3.14 / 3.95
= 1.6 s
b )
ω =

ω² = k / m
k = ω² x m
= 3.95² x .6
= 9.36 N/s
c )
v = ω
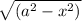
At t = .2 , x = .35
v = 3.95

= 3.95 x .357
= 1.41 m/ s
d )
Acceleration at x
a = ω² x
= 3.95 x .35
= 1.3825 m s⁻²