Question

The second sample was the same size as the first, and the proportion of sales identified as organic was 0.4. How does the 95 percent z-interval for a proportion constructed from the summer sample compare to the winter interval?

Answer 1

The summer interval is wider and has a greater point of estimate

Explanation:

Assuming this problem: "An environmental group wanted to estimate the proportion of fresh produce sales indentified as organic in a grocery store. In the winter, the group obtained a random sample of sales from the store and used the data to construct a 95percent z-interval for a proportion (0.087,0.133). Six months later in the summer, the group obtained a second random sample of sales from the store. The second sample was the same size as the first, and the proportion of sales identified as organic was 0.4. How does the 95 percent z-interval for a proportion constructed from the summer sample compare to the winter interval? "

Previous concepts

A confidence interval is "a range of values that’s likely to include a population value with a certain degree of confidence. It is often expressed a % whereby a population means lies between an upper and lower interval".

The margin of error is the range of values below and above the sample statistic in a confidence interval.

Normal distribution, is a "probability distribution that is symmetric about the mean, showing that data near the mean are more frequent in occurrence than data far from the mean".

The population proportion have the following distribution

`p \sim N(p,\sqrt{(p(1-p))/(n)})`

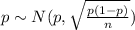
The confidence interval would be given by this formula

`\hat p \pm z_(\alpha/2) \sqrt{(\hat p(1-\hat p))/(n)}`

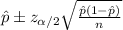
For the 95% confidence interval the value of
`\alpha=1-0.95=0.05` and
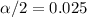`\alpha/2=0.025`, with that value we can find the quantile required for the interval in the normal standard distribution.

`z_(\alpha/2)=1.96`

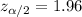
We can find the margin of error from the interval given on this case would be:

`ME= (0.133-0.087)/(2)=0.023`

And the point of estimate for the proportion obtained would be:

`\hat p = 0.133-0.023=0.11`

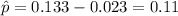
The margin of error for the proportion interval is given by this formula:

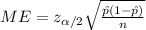`ME=z_(\alpha/2)\sqrt{(\hat p (1-\hat p))/(n)}` (a)

And on this case we have that
`ME =0.023` and we are interested in order to find the value of n, if we solve n from equation (a) we got:

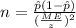`n=(\hat p (1-\hat p))/(((ME)/(z))^2)` (b)

And replacing into equation (b) the values from part a we got:

`n=(0.11(1-0.11))/(((0.023)/(1.96))^2)=710.95`

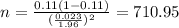
And rounded up we have that n=711

Now we know that we use the same sample size for the new confidence interval. And replacing into the confidence interval formula we got:

`0.4 - 1.96 \sqrt{(0.4(1-0.4))/(711)}=0.364`

`0.4 + 1.96 \sqrt{(0.4(1-0.4))/(711)}=0.436`

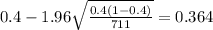
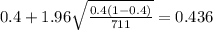
And the new 95% confidence interval would be given (0.364;0.436) has a width of 0.436-0.364=0.072. And the width for the previous confidence interval is 0.133-0.087= 0.046. So then the best answer is:

The summer interval is wider and has a greater point of estimate