Answer:
a)
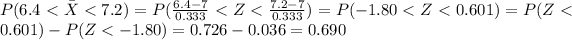
b)

So the value of bread-making machine that separates the bottom 85% of data from the top 15% is 7.345.
Explanation:
Previous concepts
Normal distribution, is a "probability distribution that is symmetric about the mean, showing that data near the mean are more frequent in occurrence than data far from the mean".
The central limit theorem states that "if we have a population with mean μ and standard deviation σ and take sufficiently large random samples from the population with replacement, then the distribution of the sample means will be approximately normally distributed. This will hold true regardless of whether the source population is normal or skewed, provided the sample size is sufficiently large".
Let X the random variable life of a bread making machine. We know from the problem that the distribution for the random variable X is given by:
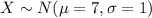
We take a sample of n=9 . That represent the sample size.
From the central limit theorem we know that the distribution for the sample mean
 is also normal and is given by:
is also normal and is given by:
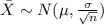

Solution to the problem
Part a
(a) the probability that the mean life of a random sample of 9 such machines falls between 6.4 and 7.2
In order to answer this question we can use the z score in order to find the probabilities, the formula given by:
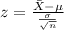
The standard error is given by this formula:
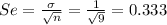
We want this probability:
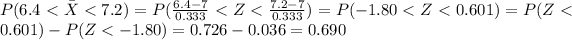
Part b
b) The value of x to the right of which 15% of the means computed from random samples of size 9 would fall.
For this part we want to find a value a, such that we satisfy this condition:
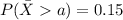 (a)
(a)
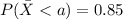 (b)
(b)
Both conditions are equivalent on this case. We can use the z score again in order to find the value a.
As we can see on the figure attached the z value that satisfy the condition with 0.85 of the area on the left and 0.15 of the area on the right it's z=1.036. On this case P(Z<1.036)=0.85 and P(Z>1.036)=0.15
If we use condition (b) from previous we have this:

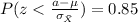
But we know which value of z satisfy the previous equation so then we can do this:
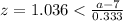
And if we solve for a we got

So the value of bread-making machine that separates the bottom 85% of data from the top 15% is 7.345.