Question

A newly issued bond pays its coupons once a year. Its coupon rate is 4.1%, its maturity is 15 years, and its yield to maturity is 7.1%.

a. Find the holding-period return for a one-year investment period if the bond is selling at a yield to maturity of 6.1% by the end of the year. (Do not round intermediate calculations. Round your answer to 2 decimal places.)
Holding-period return %
b. If you sell the bond after one year when its yield is 6.1%, what taxes will you owe if the tax rate on interest income is 40% and the tax rate on capital gains income is 30%? The bond is subject to original-issue discount (OID) tax treatment. (Do not round intermediate calculations. Round your answers to 2 decimal places.)
c. What is the after-tax holding-period return on the bond? (Do not round intermediate calculations. Round your answer to 2 decimal places.)
d. Find the realized compound yield before taxes for a two-year holding period, assuming that (i) you sell the bond after two years, (ii) the bond yield is 6.1% at the end of the second year, and (iii) the coupon can be reinvested for one year at a 2.1% interest rate. (Do not round intermediate calculations. Round your answer to 2 decimal places.)
e. Use the tax rates in part (b) to compute the after-tax two-year realized compound yield. Remember to take account of OID tax rules. (Do not round intermediate calculations. Round your answer to 2 decimal places.)

Answer 1

a) 17.53%

b) $41 x 40% = $ 16.40

815.25 - 728.48 = 86.77 capital gain x 30% = $ 26.03

Total: 26.03 + 16.40 = $ 42.43 income tax expense

c) (815.25 + 41 - 42.43) / 728.48 - 1 = 0.1171425 = 11.71%

d)

we recalculate the price of the bond with 13 years left to maturity

holding period return 26.94%

e)

tax expense:

(41x1.02 + 41) x 0.4 = 33.14

(841.87 - 728.48) x 0.3 = 34.02

tax expense: 67.16

after tax return:

(841.87 + 41x1.021 + 41 - 67.16) /728.48 - 1 = 0.177209379 = 17.72%

Step-by-step explanation:

We need to determinate the value of the bond at yield of 7.1% and at yield of 6.1% which is the sum of the present value of the maturity and coupon payment:

Purchase price:

`C * (1-(1+r)^(-time) )/(rate) = PV\\`

Coupon payment = 1,000 x 0.041 = 41.00

time 15 years

rate 0.071

`41 * (1-(1+0.071)^(-15) )/(0.071) = PV\\`

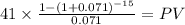
PV $371.0773

`(Maturity)/((1 + rate)^(time) ) = PV`

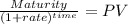
Maturity 1,000.00

time 15.00

rate 0.071

`(1000)/((1 + 0.071)^(15) ) = PV`

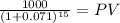
PV 357.40

PV c $ 371.0773

PV m $ 357.4028

Total $ 728.4801

Selling Price

`C * (1-(1+r)^(-time) )/(rate) = PV\\`

C 41.00

time 14 (one-year past so maturity is more closer)

rate 0.061

`41 * (1-(1+0.061)^(-14) )/(0.061) = PV\\`

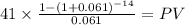
PV $378.7456

`(Maturity)/((1 + rate)^(time) ) = PV`

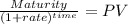
Maturity 1,000.00

time 14.00

rate 0.061

`(1000)/((1 + 0.061)^(14) ) = PV`

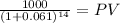
PV 436.50

PV c $378.7456

PV m $436.5004

Total $815.2460

Holding period return:

return / investment - 1

(815.25 + 41) / 728.48 - 1 = 0.175387059 = 17.53%

d)

we recalculate the price of the bond with 13 years left to maturity

`C * (1-(1+r)^(-time) )/(rate) = PV\\`

C 41.00

time 14

rate 0.061

`41 * (1-(1+0.061)^(-14) )/(0.061) = PV\\`

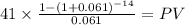
PV $378.7456

`(Maturity)/((1 + rate)^(time) ) = PV`

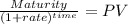
Maturity 1,000.00

time 13.00

rate 0.061

`(1000)/((1 + 0.061)^(13) ) = PV`

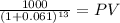
PV 463.13

PV c $378.7456

PV m $463.1269

Total $841.8725

and redo the return, tax and after-tax return:

(841.87 + 41x1.021 + 41) /728.48 - 1 = 0.269401333