Question

A recent study focused on the amount of money single men and women save monthly. The information is summarized here. Assume that the population standard deviations are equal. Sample Size Sample Mean Population Standard Deviation Men 25 23 5 Women 30 28 10 At the 0.01 significance level, what is the conclusion about the way women and men save?

Answer 1

`t=\frac{(23 -28)-(0)}{8.126\sqrt{(1)/(25)+(1)/(30)}}=-2.272`

`p_v =2*P(t_(53)<-2.272) =0.0272`

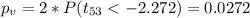
If we compare the p value obtained and using the significance level assumed
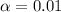`\alpha=0.01` we have
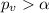`p_v>\alpha` so we can conclude that we have enough evidence to FAIL to reject the null hypothesis that the means are equal between the two groups at this significance level.

Explanation:

When we have two independent samples from two normal distributions with equal variances we are assuming that

`\sigma^2_1 =\sigma^2_2 =\sigma^2`

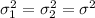
And the statistic is given by this formula:

`t=\frac{(\bar X_1 -\bar X_2)-(\mu_(1)-\mu_2)}{S_p\sqrt{(1)/(n_1)+(1)/(n_2)}}`

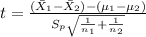
Where t follows a t distribution with
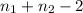`n_1+n_2 -2` degrees of freedom and the pooled variance
`S^2_p` is given by this formula:

`\S^2_p =((n_1-1)S^2_1 +(n_2 -1)S^2_2)/(n_1 +n_2 -2)`

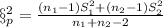
This last one is an unbiased estimator of the common variance
`\sigma^2`

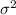
The system of hypothesis on this case are:

Null hypothesis:
`\mu_1 = \mu_2`

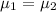
Alternative hypothesis:
`\mu_1 \\eq \mu_2`

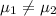
Or equivalently:

Null hypothesis:
`\mu_1 - \mu_2 = 0`

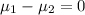
Alternative hypothesis:
`\mu_1 -\mu_2 \\eq 0`

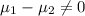
Our notation on this case :

`n_1 =25` represent the sample size for men

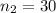`n_2 =30` represent the sample size for women

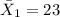`\bar X_1 =23` represent the sample mean for men

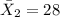`\bar X_2 =28` represent the sample mean for the women

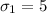`\sigma_1=5` represent the population standard deviation for men

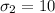`\sigma_2=10` represent the population standard deviation for women

First we can begin finding the pooled variance:

`\S^2_p =((25-1)(5)^2 +(30 -1)(10)^2)/(25 +30 -2)=66.038`

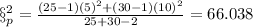
And the deviation would be just the square root of the variance:

`S_p=8.126`

And now we can calculate the statistic:

`t=\frac{(23 -28)-(0)}{8.126\sqrt{(1)/(25)+(1)/(30)}}=-2.272`

Now we can calculate the degrees of freedom given by:

`df=25+30-2=53`

And now we can calculate the p value using the altenative hypothesis:

`p_v =2*P(t_(53)<-2.272) =0.0272`

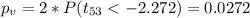
If we compare the p value obtained and using the significance level assumed
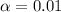`\alpha=0.01` we have
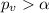`p_v>\alpha` so we can conclude that we have enough evidence to FAIL to reject the null hypothesis that the means are equal between the two groups at this significance level.