Question

An ideal diatomic gas, with rotation but no oscillation, undergoes an adiabatic compression. Its initial pressure and volume are 1.4 atm and 0.7 m3. It's final pressure is 2.1 atm. How much work is done by the gas?

Answer 1

W= -28.37 KJ

Step-by-step explanation:

Given

Gas is diatomic

We know that specific heat capacity ratio for diatomic gas ,γ = 1.4

P₁ = 1.4 atm ,V₁= 0.7 m³

P₂= 2.1 atm

Lets take final volume = V₂

We know that for adiabatic process

`P_1V_1^(\gamma)=P_2V_2^(\gamma)\\V_2=\left ((P_1)/(P_2) \right )^{(1)/(1.4)}V_1`

`V_2=\left ((1.4)/(2.1) \right )^{(1)/(1.4)}* 0.7`

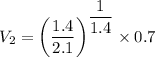
V₂ = 0.52 m³

The work done W

`W=(P_1V_1-P_2V_2)/(\gamma-1)`

`W=(1.4* 0.7-2.1* 0.52)/(1.4-1)`

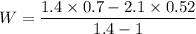
W= -0.28 atm.m³

This indicates that work is done on the gas.

W= - 0.28 atm.m³

1 atm = 101325 Pa

W= -28371 Pa.m³

W= -28.37 KJ