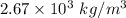Question

) A rock is suspended from a scale reads 20.0 N. A beaker of water (having a density of 1000 kg/m3) is raised up so the rock is totally submerged in the water. The scale now reads . What is the density of the rock? A) 2.50 × 103 kg/m3 B) 1.60 × 103 kg/m3 C) 2.33 × 103 kg/m3 D) 2.67 × 103 kg/m3 E) 3.00 × 103 kg/m3

Answer 1

The density of the rock is
`2.67*10^(3)\ kg/m^3`

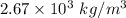
(D) is correct option.

Step-by-step explanation:

Given that,

Force = 20.0 N

Density = 1000 kg/m³

Suppose the scale new reads 12.5 N

We need to calculate the mass of rock

Using formula of force

`F= mg`

`m=(F)/(g)`

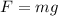
Put the value into the formula

`m=(20.0)/(10)`

`m=2\ kg`

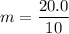
Scale reads 12.5 N after rock is totally submerged so mass of rock after submerged

We need to calculate the mass of rock

Using formula of force

`F= mg`

`m=(F)/(g)`

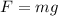
Put the value into the formula

`m=(12.5)/(10)`

`m=1.25\ kg`

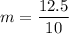
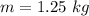
The scale reads 12.5 N = 1.25 kg so it is displacing 0.75 kg of water

We need to calculate the volume of rock using volume of water it displaces:

Using formula of volume

`Volume\ of \ rock=(0.75)/(1000)`

`V=7.5*10^(-4)`

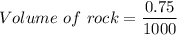
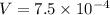
We need to calculate the density of the rock

Using formula of density

`\rho=(m)/(V)`

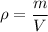
Put the value into the formula

`\rho= (2)/(7.5*10^(-4))`

`\rho=2666.66\ kg/m^3`

`\rho=2.67*10^(3)\ kg/m^3`

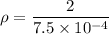
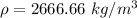
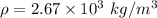
Hence, The density of the rock is
`2.67*10^(3)\ kg/m^3`