Answer:
Minimum speed will be equal to 2.213 m/sec
Step-by-step explanation:
We have given radius of the r = 2 m
Coefficient of friction
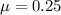
At minimum speed frictional force will be equal to centripetal force
So


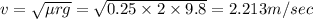
So the minimum speed will be equal to 2.213 m/sec