Question

Find the standard matrix of the linear transformation T: r^2>r^3 that first performs a counterclockwise roation by 60 degree about the origin and then reflects the result through the virticle x2 axis?

Answer 1

`A_T = \left[\begin{array}{cc}-cos(2/3 \pi)&sen(2/3 \pi)\\sen(2/3\pi)&cos(2/3\pi)\end{array}\right]`

Explanation:

60 degree is equivalent to 2/3 π. The linear transformation of a counterclockwise rotation of angle 2/3 π is

`R(2/3 \, \Pi) = \left[\begin{array}{cc}cos(2/3 \pi)&-sen(2/3 \pi)\\sen(2/3\pi)&cos(2/3\pi)\end{array}\right]`

On the other hand, the reflection throught the Y-axis is given by the linear transformation

`RY(x,y) = (-x,y)`

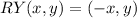
Hence its associated matrix is

`A_(RY) = \left[\begin{array}{cc}-1&0\\0&1\end{array}\right]`

And the composition is given by

`A_T = A_(RY) * R(2/3 \, \Pi) =   \left[\begin{array}{cc}-cos(2/3 \pi)&sen(2/3 \pi)\\sen(2/3\pi)&cos(2/3\pi)\end{array}\right]`